Bergische Universität Wuppertal Fachbereich Mathematik und Naturwissenschaften Angewandte Mathematik - Numerische Analysis (AMNA)

People Research Publications Teaching

---

1. Study of mathematical models of oil spills in seas
2. Numerical algorithms for transport of oil spills
3. Methods for determining the location, the time and the total power of oil emission
4. Numerical simulation of oil spills in Vietnamese coast and sea.

Summary

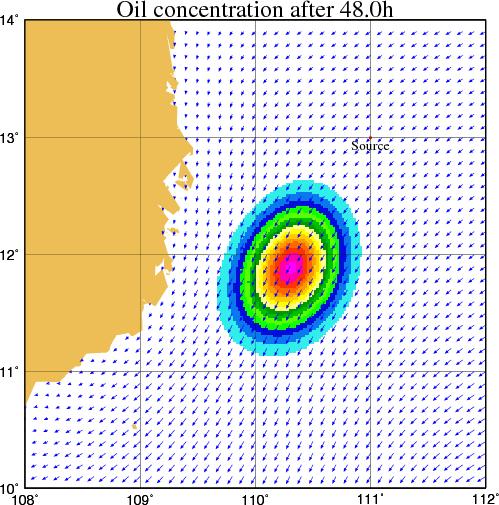
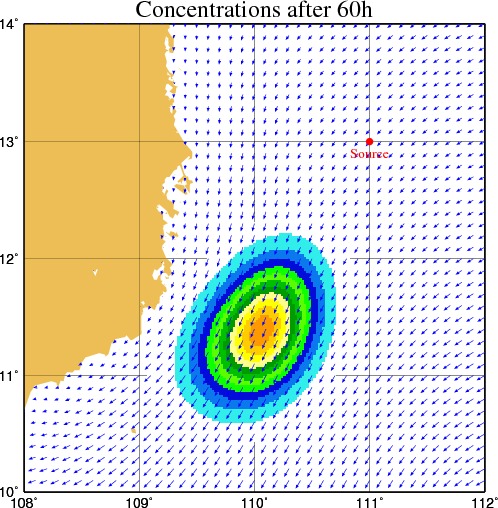
One of the goals of the present project is to study of mathematical models of oil spill processes in seas, such as advection, turbulent diffusion, surface spreading, evaporation, dissolution and emulsification. These processes may influence the transport of oil spill. Many works are focused on the surface movement of oil spills and numerical simulation of oil spills in accidents occurred in some seas on the world is published. But mathematical investigation of the computational schemes used almost is open because the authors of these works are not mathematicians. Therefore, the analysis of numerical schemes for simulating oil spills is very important. This is the first goal of the project. After completing this goal we shall obtain good numerical schemes for predicting the oil slick in any time.

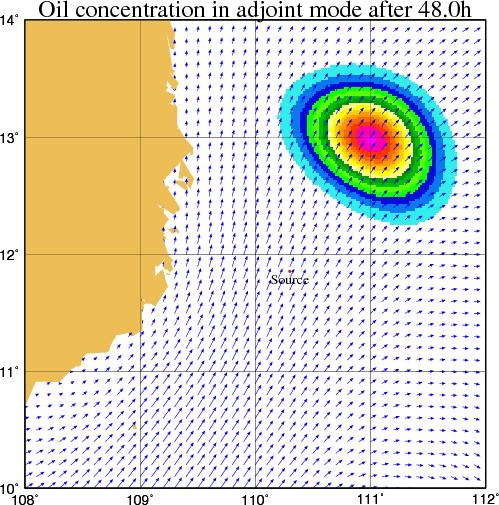
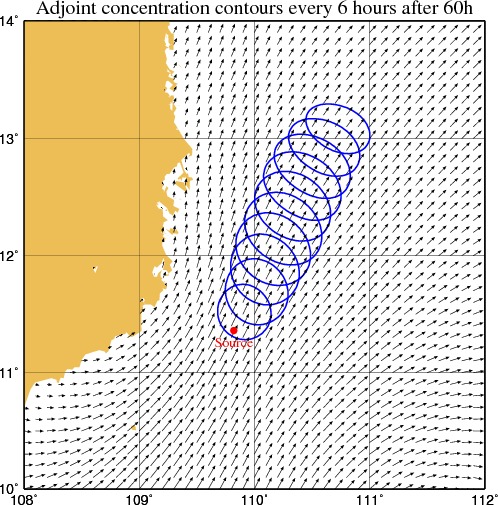
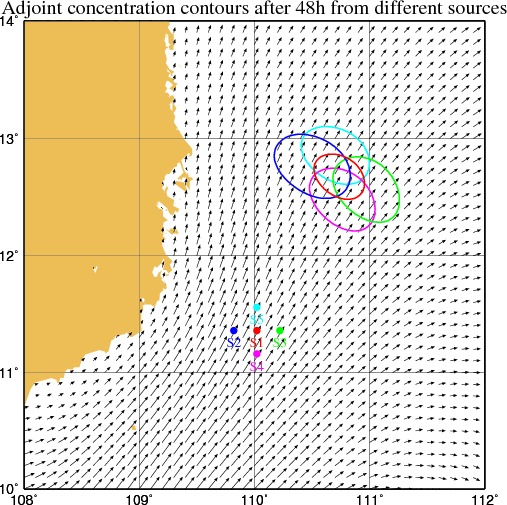
One another task, which may be more important than the prediction of oil pollution is the determination of the location, the time and the total power of oil emission if a oil slick is detected. From mathematical point of view, this is an inverse problem and it is more difficult than the direct problem of the prediction of oil pollution. So, the second goal of the project is the elaboration of methods, and numerical schemes for solving the above problem.

Of course, the above direct and inverse problems of oil spill are posed under the assumption that the wind and the flow fields in sea is known. These data in sea may be collected from experimental measurements or are obtained in the results of the problem of flow and wind.

Having in hand the data of sea flow and wind in Vietnamese coast and sea we shall simulate the spreading of oil after being discharged from a source and solve the inverse problem of determining the position, the time and the power of the source.

Simulations

German team:

• Matthias Ehrhardt (Leader)

Vietnamese team:

• Dang Quang A (Leader)
• Tran Gia Lich
• Le Duc

German institutions:

• Department of Mathematics and Informatics, Faculty of Mathematics and Natural Sciences, University of Wuppertal.

Vietnamese institutions:

• Institute of Information Technology, Hanoi
  • Department of Mathematical Methods for Information Technology
• Vietnamese Academy of Science and Technology (VAST)

Publications of the Project

[DEGD11]	Dang Quang A, M. Ehrhardt, Gia Lich Tran and Duc Le,
	Mathematical Modelling and Numerical Algorithms for Simulation of Oil Pollution, Environmental Modeling and Assessment Vol. 17, Issue 3 (2012), 275-288.
[DaEh12]	Quang A Dang and M. Ehrhardt, ,
	On Dirac delta sequences and their generating functions, Appl. Math. Lett. Vol. 25, Issue 12 (2012), 2385-2390.

Former Projects

• AIRPOLL: Mathematical Modelling and Advanced Numerical Algorithms for Simulation of Air and Water Pollution, financed by the IB of the BMBF (04/2005-07/2005)