Absorbing Boundary conditions using Titchmarsh-Weyl theory
joint work with C. Zheng, Tsinghua University, Beijing, China
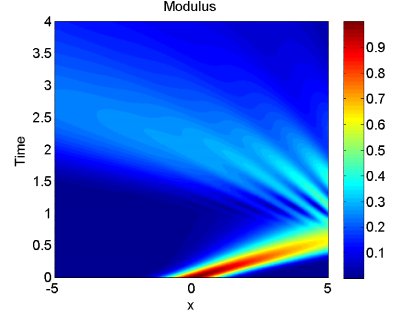
| |
Summary
A new approach for simulating the solution of the time-dependent
Schrödinger equation with a general variable potential will be
proposed. The key idea is to approximate the Titchmarsh-Weyl
m-function (exact Dirichlet-to-Neumann operator) by a rational
function with respect to the spectral parameter.
With the proposed method
we can overcome the usual high-frequency restriction for absorbing
boundary conditions of general variable potential problems.
We end up with a fast computational algorithm for absorbing
boundary conditions that are accurate for the full frequency band.
Application Partners
References
- X. Antoine, A. Arnold, C. Besse, M. Ehrhardt and A. Schädle,
A Review of Transparent and Artificial Boundary Conditions Techniques for Linear and Nonlinear Schrödinger Equations,
Commun. Comput. Phys. 4 (2008), 729-796. (open-access article)
supplementary material (Matlab codes):
- M. Ehrhardt and C. Zheng,
Implementing exact absorbing boundary condition for the linear one-dimensional
Schrödinger problem with variable potential by Titchmarsh-Weyl theory,
WIAS Preprint No. 1426, July 2009 (submitted to: Journal of Computational Physics)




